SECTION – I
(4 * 1 = 4)
Question 1: Write A = {2, 4, 8, 16} in set-builder form.
Solution:
A = {2n / n ∈ N, and n < 5}
Question 2: Find the value of log5 √625.
Solution:
log5 √625
= log5 25
= log5 52
= 2 log5 5
= 2 * 1
= 2
Question 3: The larger of two supplementary angles exceeds the smaller by 58o, then find the angles.
Solution:
Let the required supplementary angles be x and y.
x + y = 180o —- (1)
The larger angle exceeds the smaller by 58o.
x – y = 58o —- (2)
Solve (1) and (2),
2x = 238
x = 238 / 2
x = 119o
y = 61o
Question 4: Find the curved surface area of the cylinder, whose radius is 7cm and height is 10cm.
Solution:
Radius if the cylinder (r) = 7cm
Height of the cylinder (h) = 10cm
The curved surface area of the cylinder = 2𝛑rh
= 2 * (22 / 7) * (7) * (10)
= 440 cm2
SECTION – II
(5 * 2 = 10)
Question 5: Rohan’s mother is 26 years older than him. The product of their ages after 3 years will be 360. Then write the required quadratic equation to find Rohan’s present age.
Solution:
Let Rohan’s present age be x years.
His mother’s age at present is (x + 26) years.
After 3 years, Rohan’s age = (x + 3) years
After 3 years, his mother’s age = (x + 26) + 3 = (x + 29) years
The product of their ages = (x + 3) (x + 29)
= x * x + x * 29 + 3 * x + 3 * 29
= x2 + 29x + 3x + 87
= x2 + 32x + 87
By the sum, the product of their ages is 360
x2 + 32x + 87 = 360
x2 + 32x + 87 – 360 = 0
x2 + 32x – 273 = 0 is the required quadratic equation.
Question 6: Find the zeroes of the quadratic polynomial x2 – x – 30 and verify the relation between the zeroes and its coefficients.
Solution:
Given the polynomial x2 – x – 30,
To find the zeros, x2 – x – 30 = 0 [say]
= x2 – 6x + 5x – 30
= x (x – 6) + 5 (x – 6)
= (x + 5) (x – 6)
x = -5 and x = 6
Sum of the zeroes = 6 + (-5)
= 1
= (-1) / 1
= – (coefficient of x) / (coefficient of x2)
Product of the zeroes = 6 (-5)
= -30
= (-30 / 1)
= (constant term) / (coefficient of x2)
Question 7: A joker’s cap is in the form of a right circular cone, whose base radius is 7cm and height is 24cm. Find the area of the sheet required to make 10 such caps.
Solution:
Base radius of the conical cap (r) = 7cm
Height (h) = 24cm
Slant height (l) = √r2 + h2
= √72 + 242
= √49 + 576
= √625
= 25 cm
Area of sheet required to make a cap = Lateral surface area of the cap
= 𝛑rl
= (22 / 7) * 7 * 25
= 550 sq.cm
Area of sheet required to 10 such caps
= 10 * 550
= 5500 sq.cm
Question 8: Find the HCF of 1260 and 1440 by using Euclid’s division lemma.
Solution:
The given numbers are 1260 and 1440.
1440 = 1260 * 1 + 180
1260 = 180 * 7 + 0
HCF of 1440 and 1260 is 180.
Question 9: If the sum of the first 15 terms of an AP is 675 and its first term is 10, then find 25th term.
Solution:
First-term of an AP = a = 10
Let the common difference be d.
Sum of the first 15 terms is S15 = 675
(15 / 2) = [2a + 14d] = 675
[2 * 10] + 14d = (675 * 2) / 15
14d = 90 – 20 = 70
d = 70 / 14
d = 5
25th term of an AP is a25 = a + 24d
= 10 + 24 * 5
= 10 + 120
= 130
SECTION – III
(4 * 4 = 16)
Question 10:
[a] Show that 2 + 5√3 is irrational.
OR
[b] Check whether -321 is a term of the AP 22, 5, 8, 1…..
Solution:
[a] Let us assume the contrary that 2 + 5√3 is rational that is coprime can be found for ‘a’ and ‘b’ and b ≠ 0 such that
2 + 5√3 = (a / b)
5√3 = (a / b) – 2
√3 = (a / 5b) – (2 / 5)
Since (a / 5b) and (2 / 5) ∈ Q, (a / 5b) – (2 / 5) ∈ Q.
So, √3 is rational.
But this contradicts the fact that √3 is irrational.
So, our assumption that 2 + 5√3 is rational is wrong.
So, 2 + 5√3 is irrational.
[b] From the given AP, 22, 15, 8, 1 …..
a = 22, d = -7
nth term of an AP = an = a + (n – 1)d
In this AP, let the nth term be -321
a + (n – 1)d = -321
22 + (n – 1) (-7) = -321
(n – 1) (-7) = -343
n – 1 = (-343) / (-7)
n = 49 + 1
n = 50
Hence, -321 will be the 50th term in the given AP.
Question 11:
[a] In a class test, the sum of Moulika’s marks in mathematics and English is 30. If she got 2 marks more in mathematics and 3 marks less in English, the product of her marks would have been 210. Find her marks in the two subjects.
OR
[b] An oil drum is in the shape of the cylinder, whose diameter is 2m and height is 7m. The painter charges Rs. 5 per m2 to paint the drum. Find the total charges to be paid to the painter for 10 drums.
Solution:
[a] Given that the sum of Moulika’s marks in Mathematics and English is 30.
Let the marks of Moulika in Mathematics be x and that of in English be 30 – x.
If she got 2 marks more in mathematics then marks in maths = x + 2
And she got 3 marks less in English then the marks in English = 30 – x – 3 = 27 – x
Product of these two = (x + 2) (27 – x) = 210
x2 – 25x + 156 = 0
(x – 12) (x – 13) = 0
x = 12, 13
Case (i) If x = 12, the marks of Moulika in
Mathematics = 12
English = 30 – 12 = 18
Case (ii) If x = 13, the marks of Moulika in
Mathematics = 13
English = 30 – 13 = 17
[b] The diameter of the oil drum which is in the shape of cylinder = d = 2m
The radius of the drum = r = d / 2 = 2 / 2 = 1m
Height = h = 7cm
Total surface area of the drum which is in the shape of cylinder = 2𝛑r (r + h)
= 2 * (22 / 7) * (1) * (1 + 7)
= 2 * (22 / 7) * 8
= 50.28 sq.m
Charges to paint the drum per sq.m = Rs. 5
The total cost of painting 10 such type of drums = 50.28 * 5 * 10 = Rs. 2514
Question 12:
(i) [a] If A = {x : x is a natural number less than is 6}.
B = {x : x is a prime number which is a divisor of 60}.
C = {x : x is an odd natural number less than 10}.
D = {x : x is an even natural number which is a divisor of 48}.
Then write the roster form for all the above sets and find
[a] A ⋃ B
[b] B ⋂ C
[c] A – D
[d] D – B
OR
(ii) 6 pencils and 4 notebooks together cost Rs. 90 whereas 8 pencils and 3 notebooks together cost Rs. 85. Find the cost of one pencil and that of one notebook.
Solution:
(i) [a] A = {1, 2, 3, 4, 5}
B = {2, 3, 5}
C = {1, 3, 5, 7, 9}
D = {2, 4, 6, 8, 12, 14, 16, 24, 48}
[a] A ⋃ B = {1, 2, 3, 4, 5} ⋃ {2, 3, 5} = {1, 2, 3, 4, 5}
[b] B ⋂ C = {2, 3, 5} ⋂ {1, 3, 5, 7, 9} = {3, 5}
[c] A – D = {1, 2, 3, 4, 5} – {2, 4, 6, 8, 12, 14, 16, 24, 48} = {1, 3, 5}
[d] D – B = {2, 4, 6, 8, 12, 14, 16, 24, 48} – {2, 3, 5} = {4, 6, 8, 12, 16, 24, 48}
(ii) Let the cost of one pencil be Rs. x.
Cost of one notebook = Rs. y.
The total cost of 6 pencils and 4 notebooks = Rs. 90
6x + 4y = 90 —- (1)
The total cost of 8 pencils and 3 notebooks = Rs. 85
8x + 3y = 85 —- (2)
On solving the above two equations, x = 5, y = 15.
The cost of one pencil = Rs. 5
The cost of one notebook = Rs. 15
Question 13:
[a] Find the zeroes of the quadratic polynomial p(x) = x2 + x – 20 using the graph.
OR
[b] Solve the following pair of linear equations graphically.
2x + y + 4 and 2x – 3y = 12.
Solution:
[a] Let y = x2 + x – 20

[b]

SECTION – IV
(20 * 0.5 = 10)
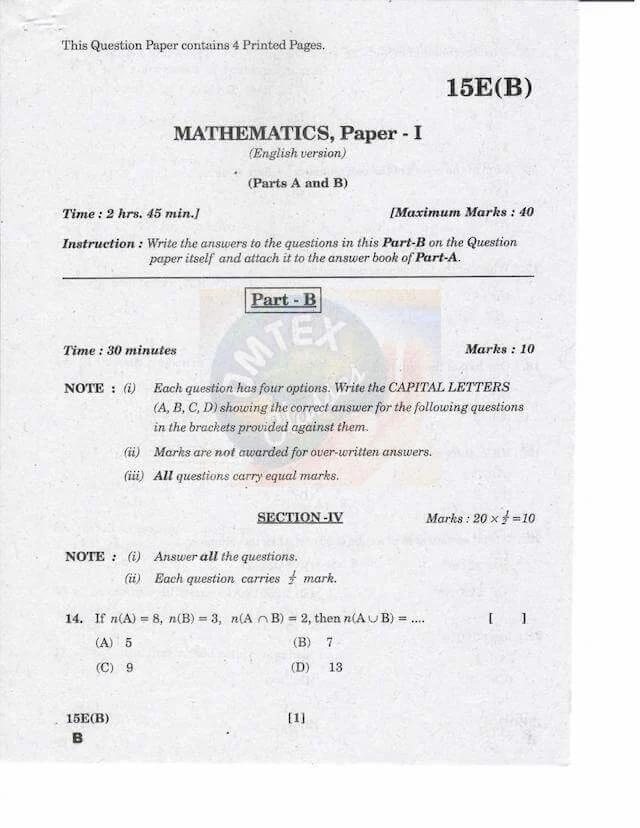
Question 14: If n(A) = 8, n (B) = 3, n (A ⋂ B) = 2, then n (A ⋃ B) = ______
(A) 5 (B) 7 (C) 9 (D) 13
Answer: C
Question 15: The discriminant of 6x2 – 5x + 1 = 0 is ______
(A) 1 (B) 2 (C) 6 (D) (-5 / 6)
Answer: A
Question 16: Sum of the zeroes of the polynomial x2 + 5x + 6 = 0 is
(A) 5 (B) -5 (C) 6 (D) (5 / 6)
Answer: B
Question 17: Which of the following is not irrational?
(A) √2 (B) √3 (C) √4 (D) √5
Answer: C
Question 18: One root of the equation x – (3 / x) = 2 is _____
(A) 1 (B) 2 (C) 3 (D) 4
Answer: C
Question 19: If 4, a, 9 are in GP, then a = ____
(A) 6 (B) ±6 (C) 7 (D) ±7
Answer: B
Question 20: If the total surface area of a cube is 96cm2, then its volume is _____
(A) 32cm3 (B) 64 cm3 (C) 128cm3 (D) 256 cm3
Answer: B
Question 21: log 10 0.001 = _____
(A) 2 (B) 3 (C) -2 (D) -3
Answer: D
Question 22: Match the following.
If a, b, c are the zeroes of a cubic polynomial ax3 + bx2 + cx + d = 0, then
[i] a + b + c [a] (-d / a)
[ii] ab + bc + ca [b] (c / a)
[iii] abc [c] (-b / a)
(A) [i] – c, [ii] – b, [iii] – a
(B) [i] – a, [ii] – b, [iii] – c
(C) [i] – b, [ii] – a, [iii] – c
(D) [i] – c, [ii] – a, [iii] – b
Answer: A
Question 23: The next term in AP √3, √12, √27, ……
(A) √32 (B) √36 (C) √42 (D) √48
Answer: D
Question 24: The shaded region in the figure shows

(A) A – B (B) B – A (C) μ – B (D) A ⋃ B
Answer: C
Question 25: 5x – 3 represents ______ polynomial.
(A) Linear (B) Quadratic (C) Cubic (D) Fourth degree
Answer: A
Question 26: The common difference in AP log2 2, log2 4, log2 8 …… is
(A) 1 (B) 2 (C) 3 (D) 4
Answer: A
Question 27: The sum of the first ‘n’ odd natural numbers is ______
(A) n (B) n2 (C) n (n + 1) (D) n (n + 1) / 2
Answer: B
Question 28: The quadratic polynomial, whose zeroes are √2 and √-2 is ____
(A) x2 – 2 (B) x2 + 2 (C) x2 + √2 (D) x – 2
Answer: A
Question 29: The number of zeroes of the polynomial in the graph is _____

(A) 0 (B) 1 (C) 2 (D) 3
Answer: D
Question 30: The line 2x – 3y = 8 intersects x-axis at
(A) (2, -3) (B) (0, -3) (C) (2, 0) (D) (4, 0)
Answer: D
Question 31: The volume of the cone, whose radius is 3cm and height is 8cm, is _____ cm3.
(A) 6𝛑 (B) 12𝛑 (C) 18𝛑 (D) 24𝛑
Answer: D
Question 32: If 6x + 2y + 9 = 0 and kx + y – 7 = 0 has no solution, then k = ______
(A) 3 (B) 2 (C) -3 (D) -2
Answer: A
Question 33: If the equation x2 + 5x + k = 0 has real and distinct roots, then ____
(A) k = 6 (B) k < 6.25 (C) k > 6 (D) k > 25
Answer: B