Mathematical Logic Exercise 1.5 [Page 12] Balbharati solutions for Mathematics and Statistics 1 (Commerce) 12th Standard HSC Maharashtra State Board Chapter 1

EXERCISE 1.5PAGE 12

Balbharati solutions for Mathematics and Statistics 1 (Commerce) 12th Standard HSC Maharashtra State Board Chapter 1 Mathematical Logic Exercise 1.5 [Page 12]

EXERCISE 1.5Q 1.1    PAGE 12

Exercise 1.5 | Q 1.1 | Page 12

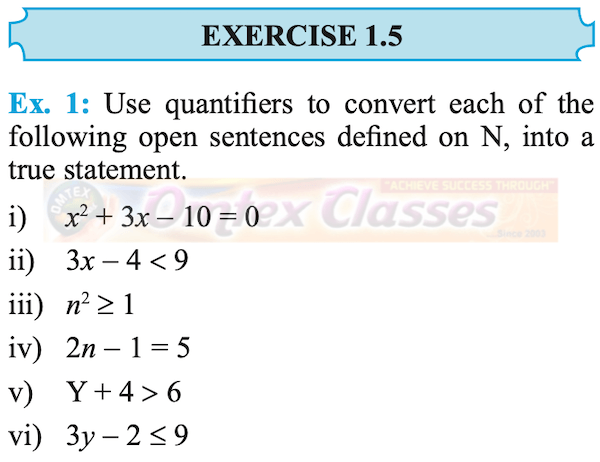
Use quantifiers to convert the following open sentences defined on N, into a true statement.

x² + 3x - 10 = 0 

SOLUTION

∃ x ∈ N, such that x² + 3x – 10 = 0

It is true statement, since x = 2 ∈ N satisfies it.

EXERCISE 1.5Q 1.2   PAGE 12

Exercise 1.5 | Q 1.2 | Page 12

Use quantifiers to convert the following open sentences defined on N, into a true statement.

3x - 4 < 9

SOLUTION

∃ x ∈ N, such that 3x – 4 < 9

It is true statement, since

x = 2, 3, 4 ∈ N satisfies 3x - 4 < 9.

EXERCISE 1.5Q 1.3    PAGE 12

Exercise 1.5 | Q 1.3 | Page 12

Use quantifiers to convert the following open sentences defined on N, into a true statement.

n² ≥ 1

SOLUTION

∀ n ∈ N, n² ≥ 1

It is true statement, since all n ∈ N satisfy it.

EXERCISE 1.5Q 1.4    PAGE 12

Exercise 1.5 | Q 1.4 | Page 12

Use quantifiers to convert the following open sentences defined on N, into a true statement.

2n - 1 = 5

SOLUTION

∃ n ∈ N, such that 2n - 1 = 5

It is a true statement since all n = 3 ∈ N satisfy 2n - 1 = 5.

EXERCISE 1.5Q 1.5    PAGE 12

Exercise 1.5 | Q 1.5 | Page 12

Use quantifiers to convert the following open sentences defined on N, into a true statement.

y + 4 > 6

SOLUTION

∃ y ∈ N, such that y + 4 > 6

It is a true statement since y = 3, 4, ... ∈ N satisfy y + 4 > 6.

EXERCISE 1.5Q 1.6    PAGE 12

Exercise 1.5 | Q 1.6 | Page 12

Use quantifiers to convert the following open sentences defined on N, into a true statement.

3y - 2 ≤ 9

SOLUTION

∃ y ∈ N, such that 3y - 2 ≤ 9

It is a true statement since y = 1, 2, 3 ∈ N satisfy it.

EXERCISE 1.5Q 2.1    PAGE 12

Exercise 1.5 | Q 2.1 | Page 12

If B = {2, 3, 5, 6, 7} determine the truth value of ∀ x ∈ B such that x is prime number.

SOLUTION

For x = 6, x is not a prime number.

∴ x = 6 does not satisfies the given statement.

∴ The given statement is false.

∴ It’s truth value is F.

EXERCISE 1.5Q 2.2    PAGE 12

Exercise 1.5 | Q 2.2 | Page 12

If B = {2, 3, 5, 6, 7} determine the truth value of
∃ n ∈ B, such that n + 6 > 12.

SOLUTION

For n = 7, n + 6 = 7 + 6 = 13 > 12

∴ n = 7 satisfies the equation n + 6 > 12.

∴ The given statement is true.

∴ It’s truth value is T.

EXERCISE 1.5Q 2.3    PAGE 12

Exercise 1.5 | Q 2.3 | Page 12

If B = {2, 3, 5, 6, 7} determine the truth value of
∃ n ∈ B, such that 2n + 2 < 4.

SOLUTION

There is no n in B which satisfies 2n + 2 < 4.

∴ The given statement is false.

∴ It’s truth value is F.

EXERCISE 1.5Q 2.4    PAGE 12

Exercise 1.5 | Q 2.4 | Page 12

If B = {2, 3, 5, 6, 7} determine the truth value of
∀ y ∈ B, such that y² is negative.

SOLUTION

There is no y in B which satisfies y² < 0.

∴ The given statement is false.

∴ It’s truth value is F.

EXERCISE 1.5Q 2.5    PAGE 12

Exercise 1.5 | Q 2.5 | Page 12

If B = {2, 3, 5, 6, 7} determine the truth value of
∀ y ∈ B, such that (y - 5) ∈ N

SOLUTION

For y = 2, y – 5 = 2 – 5 = –3 ∉ N.

∴ y = 2 does not satisfies the equation (y – 5) ∈ N.

∴ The given statement is false.

∴ It’s truth value is F.