Subtraction: Concepts, Strategies, and Applications
Three sources explore subtraction's significance, encompassing its core concepts, diverse teaching strategies, and real-world applications. Visual aids, such as ten frames and number lines, are highlighted as crucial for effective learning. The sources detail various methods, including counting back, using objects, and employing fact families, to build subtraction proficiency. Furthermore, they emphasize subtraction's role in problem-solving, critical thinking, and daily life, underscoring its importance beyond basic mathematics. Mastering subtraction is presented as foundational for future mathematical success.
Subtraction: Strategies and Applications
Subtraction: A Multifaceted Learning Journey
This briefing document reviews the main themes and key takeaways from three provided sources on subtraction. The sources, "Subtraction Strategies and Applications," "Subtraction Strategies: A Comprehensive Guide," and "Testing Theme: Subtraction.pdf," collectively illuminate the importance of subtraction, its practical applications, and various strategies to facilitate its comprehension.
Core Concepts of Subtraction
Subtraction, the inverse of addition, involves finding the difference between two numbers – the minuend (starting number) and the subtrahend (the number being subtracted). As emphasized in both "Subtraction Strategies and Applications" and "Subtraction Strategies: A Comprehensive Guide," understanding these basic components is crucial for grasping the essence of subtraction.
Visualization: A Key to Understanding
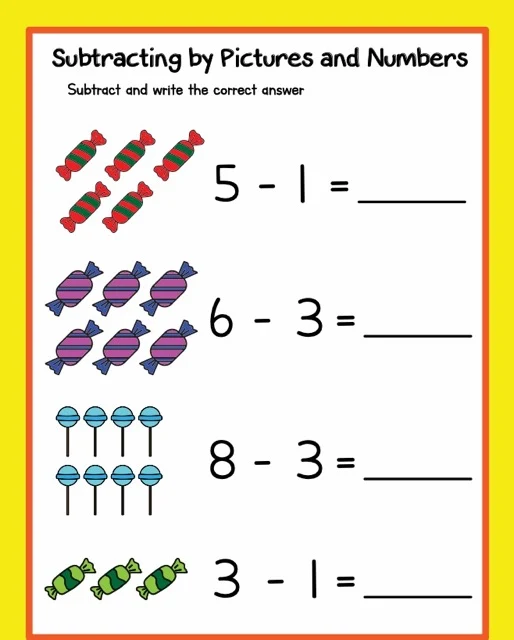
Both textual sources highlight the significance of visualization in teaching and learning subtraction. "Subtraction Strategies and Applications" states that "Pictures are great visual aids for understanding subtraction." The use of ten frames and number lines is repeatedly emphasized as effective visual tools. "Testing Theme: Subtraction.pdf" provides practical examples of using ten frames for subtraction exercises, reinforcing the practical application of this concept.
Strategies for Effective Learning
The sources detail numerous strategies to aid subtraction learning:
●
Counting Back: Starting at the minuend and counting backward by the subtrahend. ("Subtraction Strategies: A Comprehensive Guide" explains, "For instance, to solve 7 - 2, you begin at 7 and count backward two numbers: 6, 5. Therefore, 7 - 2 = 5.")
●
Using Objects: Utilizing physical or visual representations to depict the subtraction process.
●
Ten Frames: Employing grids to visually represent numbers and the act of taking away.
●
Number Lines: Moving leftward on the number line to determine the difference.
●
Making Ten: Breaking down the subtrahend to utilize the number ten as a stepping stone.
●
Fact Families: Recognizing the relationship between addition and subtraction facts.
Real-World Relevance and Importance
All three sources stress the pervasiveness of subtraction in everyday scenarios. "Subtraction Strategies and Applications" provides examples like sharing cookies, spending money, and calculating time. "Subtraction Strategies: A Comprehensive Guide" further emphasizes the application in shopping, baking, and financial management.
Mastering subtraction proves crucial for advanced mathematical concepts, such as multiplication, division, and algebra. It equips individuals with essential skills for:
●
Problem-solving: Subtraction problems encourage analytical and logical thinking. ("Subtraction problems often require logical thinking, strategic approaches, and the ability to break down complex situations into smaller, solvable steps," as stated in "Subtraction Strategies: A Comprehensive Guide.")
●
Critical Thinking: The ability to deconstruct complex scenarios into manageable components.
●
Decision Making: Subtraction aids informed decision-making in diverse situations.
Conclusion
The reviewed sources underscore the foundational importance of subtraction, not just within mathematics but also in daily life. The emphasis on diverse learning strategies, particularly visual aids like ten frames and number lines, highlights the need for adaptable teaching approaches. The integration of real-world examples further reinforces the practical value of subtraction, motivating learners to grasp this essential mathematical skill.
Subtraction Strategies and Applications
Subtraction FAQ
1. What is subtraction?
Subtraction is a mathematical operation where you take away a certain number (the subtrahend) from another number (the minuend) to find the difference. It is the opposite of addition.
2. How can I use pictures to help me subtract?
Pictures are great visual aids for understanding subtraction. You can start with a certain number of objects, then cross out or remove the number you want to subtract. The remaining objects represent the difference.
3. What are ten frames, and how can they be used for subtraction?
Ten frames are rectangular grids divided into ten equal spaces. They help visualize numbers up to ten. To subtract using ten frames, you fill the frame with counters representing the minuend, then remove the number of counters indicated by the subtrahend. The remaining counters represent the difference.
4. What are number lines, and how can they be used for subtraction?
A number line is a line where numbers are marked at equal intervals. To subtract using a number line, start at the minuend and then move to the left the number of spaces indicated by the subtrahend. The number you land on represents the difference.
5. What are some real-life examples of subtraction?
Subtraction is used in countless everyday situations:
●
Sharing: If you have five cookies and give two to a friend, you are left with three cookies (5 - 2 = 3).
●
Spending Money: If you have $10 and buy a snack for $3, you are left with $7 ($10 - $3 = $7).
●
Time: If a movie starts at 7:00 pm and lasts for two hours, it will end at 9:00 pm (7 + 2 = 9).
6. What are some strategies for solving subtraction problems?
●
Counting Back: Start with the minuend and count backward the number of times indicated by the subtrahend.
●
Using Objects: Utilize physical objects like counters or your fingers to represent the numbers and physically remove the subtrahend to find the difference.
●
Using Ten Frames: Visualize the subtraction process within a ten frame, removing counters to find the difference.
●
Using Number Lines: Move leftward on a number line, starting from the minuend, to determine the difference.
7. How is subtraction related to addition?
Subtraction is the inverse operation of addition. This means that subtraction "undoes" addition. For example, if you add 3 to 5 to get 8 (5 + 3 = 8), you can then subtract 3 from 8 to get back to 5 (8 - 3 = 5).
8. Why is it important to learn subtraction?
Subtraction is a foundational mathematical skill essential for:
●
Higher-level math: Understanding subtraction is crucial for learning multiplication, division, and algebra.
●
Everyday life: We use subtraction constantly for managing money, telling time, and solving various practical problems.
●
Problem-solving and critical thinking: Subtraction encourages logical thinking and problem-solving skills that are valuable in many areas of life.
Subtraction Strategies: A Comprehensive Guide
Subtraction Strategies: A Review
Subtraction Strategies FAQ
1. What are the key components of a subtraction problem?
Subtraction problems involve three key components: the minuend, which is the starting number; the subtrahend, which is the number being subtracted; and the difference, which is the result of the subtraction. For example, in the equation 8 - 3 = 5, 8 is the minuend, 3 is the subtrahend, and 5 is the difference.
2. Describe the "counting back" method for subtraction.
The "counting back" method is a basic subtraction strategy where you start with the minuend and count backward a number of times equal to the subtrahend. For instance, to solve 7 - 2, you begin at 7 and count backward two numbers: 6, 5. Therefore, 7 - 2 = 5.
3. How do ten frames help in visualizing subtraction?
Ten frames are rectangular grids with ten spaces, useful for visualizing numbers up to ten. For subtraction, you fill the frame with counters representing the minuend, then remove counters equal to the subtrahend. The leftover counters show the difference.
4. Explain the use of number lines for subtraction.
A number line is a line with numbers at equal intervals. To subtract, you start at the minuend on the number line and move left a number of spaces equal to the subtrahend. The number you land on is the difference.
5. Provide two real-life examples where subtraction is applied.
●
Shopping: If you have $20 and spend $8 on groceries, you can subtract to find your remaining balance: $20 - $8 = $12.
●
Baking: If a recipe requires 8 cups of flour and you only have 3, subtracting helps determine how much more you need: 8 - 3 = 5 cups.
6. How does the concept of inverse operations relate addition and subtraction?
Addition and subtraction are inverse operations, meaning they reverse each other. If you add a number and then subtract the same number, you return to the original value. For example, 5 + 3 = 8, and 8 - 3 = 5.
7. What is the significance of mastering subtraction for advanced math?
Understanding subtraction is crucial for more complex mathematical concepts like multiplication, division, and algebra. These operations often build upon and incorporate subtraction principles.
8. What are the benefits of learning subtraction in everyday situations?
Subtraction is constantly used in daily life for tasks like managing finances, calculating time differences, and making informed decisions involving quantities.
9. Describe the role of subtraction in problem-solving and critical thinking.
Subtraction problems often require logical thinking, strategic approaches, and the ability to break down complex situations into smaller, solvable steps, fostering critical thinking skills.
10. Beyond counting back, objects, and ten frames, what other strategies can help with subtraction?
Other strategies include: * Making ten: Subtracting a number from 10 can be easier, so you can break down the subtrahend. For example, for 12 - 5, think of it as 12 - 2 - 3, using 10 as a stepping stone. * Fact families: Recognizing that addition and subtraction facts are related can help. If you know 4 + 3 = 7, you also know 7 - 3 = 4 and 7 - 4 = 3.
Short-Answer Quiz
Instructions: Answer the following questions in 2-3 sentences each.
1.
What is the role of the minuend in a subtraction problem?
2.
Explain how you would use the "using objects" strategy to solve 9 - 4.
3.
In the context of a ten frame, what does the difference represent after subtraction?
4.
If you have 6 apples and give 2 to a friend, how can you represent this situation using a number line?
5.
Describe a real-life scenario where you would need to use subtraction to solve a problem.
6.
How does knowing that 3 + 5 = 8 help you solve 8 - 5?
7.
Why is understanding subtraction important for learning multiplication?
8.
Give an example of how subtraction is used in managing personal finances.
9.
Explain how subtraction problems can enhance logical thinking skills.
10.
Besides the strategies mentioned in the FAQ, name one other method for solving subtraction problems.
Answer Key
1.
The minuend is the starting number in a subtraction problem. It represents the total amount from which another quantity (the subtrahend) will be taken away.
2.
To solve 9 - 4 using objects, you would start with 9 objects (like blocks or counters). Then, remove 4 of the objects. The number of objects remaining (5) is the difference.
3.
In a ten frame, after removing counters representing the subtrahend, the remaining counters represent the difference between the minuend and the subtrahend.
4.
On a number line, you would start at the number 6. Then, move two spaces to the left (since you are giving away 2 apples). The number you land on, 4, represents the number of apples remaining.
5.
If you are at the grocery store with $25 and want to buy items that total $18, you need to use subtraction to calculate how much money you will have left after the purchase ($25 - $18 = $7).
6.
Knowing that 3 + 5 = 8 reveals a fact family. Since addition and subtraction are inverse operations, knowing this addition fact automatically tells you that 8 - 5 = 3.
7.
Multiplication can be understood as repeated addition. For example, 4 x 3 is the same as 3 + 3 + 3 + 3. Subtraction helps in understanding these groupings and in solving multiplication problems involving remainders or partial products.
8.
When balancing a checkbook, you subtract the amounts of checks written and debit card purchases from the starting balance to keep track of the remaining funds in the account.
9.
Subtraction problems require analyzing the given information, determining the appropriate operation, and applying the subtraction process correctly. This sequence of steps fosters logical thinking and helps develop problem-solving strategies.
10.
The "making ten" strategy can be helpful. For instance, for 15 - 8, you can think of it as 15 - 5 - 3. Subtracting 5 from 15 gets you to 10, and then subtracting the remaining 3 gives you the answer, 7.
Essay Questions
1.
Discuss the importance of using multiple strategies to teach subtraction to young learners. How can different approaches cater to diverse learning styles and enhance understanding?
2.
Analyze the relationship between addition and subtraction as inverse operations. How does this concept contribute to a deeper understanding of both operations?
3.
Compare and contrast the use of ten frames and number lines as visual aids for subtraction. What are the strengths and limitations of each tool, and in what situations might one be more beneficial than the other?
4.
Explore the role of subtraction in real-world problem-solving. Provide specific examples from various fields, such as finance, time management, or measurement, and explain how subtraction is applied to find solutions.
5.
How can technology be effectively integrated into teaching and learning subtraction? Discuss specific digital tools or resources and explain how they can enhance student engagement, provide personalized learning experiences, and support the development of subtraction skills.
Glossary of Key Terms
Minuend: The starting number in a subtraction problem; the number from which another number is subtracted.
Subtrahend: The number being subtracted from the minuend.
Difference: The result of a subtraction problem.
Counting Back: A subtraction strategy where you start with the minuend and count backward a number of times equal to the subtrahend.
Ten Frame: A rectangular grid with ten spaces that helps visualize numbers and perform operations like subtraction.
Number Line: A line with numbers marked at equal intervals, used for various mathematical operations, including subtraction.
Inverse Operations: Operations that reverse each other, like addition and subtraction.
Fact Family: A group of related addition and subtraction facts that use the same numbers. For example: 3 + 5 = 8, 5 + 3 = 8, 8 - 3 = 5, 8 - 5 = 3.